According to the definition of subgradient
$$ \mathcal{L}(\mathbf{u}) \geq \mathcal{L}(\mathbf{w})+\mathbf{g}^{\top}(\mathbf{u}-\mathbf{w}) \quad \forall \mathbf{u} $$
Could we define the following gradients? A. From [-2,0] It is touching the function and it is bellow the function
B. From [0,1] It is touching the function and it is bellow the function
Thank you in advance :)
these look reasonable only in a local neighborhood, but they do not satisfy the actual subgradient definition you stated (the 'for all u' part is meant global)
Thank you very much for answering :)
In this case, only x = 2 and x = 3 have a subgradient because it is the only subgradient that is globally bellow the function for [-2,4]?
In the following function, can we say that the function has a subgradient with value 2 at x = 0, x = 1, x = 2 and x = 3?
yes, and yes
Subgradients
According to the definition of subgradient
$$ \mathcal{L}(\mathbf{u}) \geq \mathcal{L}(\mathbf{w})+\mathbf{g}^{\top}(\mathbf{u}-\mathbf{w}) \quad \forall \mathbf{u} $$
Could we define the following gradients?
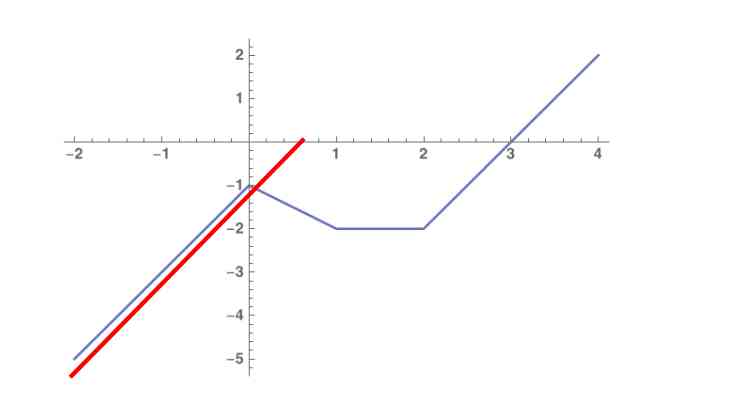
A. From [-2,0]
It is touching the function and it is bellow the function
B. From [0,1]
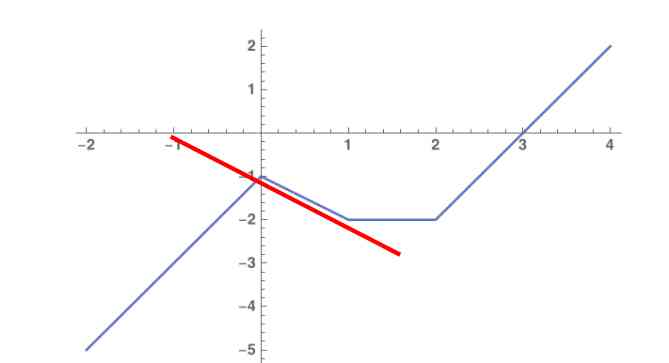
It is touching the function and it is bellow the function
Thank you in advance :)
1
these look reasonable only in a local neighborhood, but they do not satisfy the actual subgradient definition you stated (the 'for all u' part is meant global)
2
Thank you very much for answering :)
In this case, only x = 2 and x = 3 have a subgradient because it is the only subgradient that is globally bellow the function for [-2,4]?
In the following function, can we say that the function has a subgradient with value 2 at x = 0, x = 1, x = 2 and x = 3?
yes, and yes
1
Add comment